Are you tired of making errors when calculating percentages? Do you struggle with calculating percentage relative error? If so, you are not alone. It can be frustrating to make these mistakes, especially when they could have been easily avoided.
Many people struggle with calculating percentage relative error, whether it’s for school, work, or personal use. It can be confusing to know where to start or what formula to use. This can cause stress and delay in completing tasks.
If you want to make sure that you’re getting accurate calculations every time, it’s important to understand the basics of how to calculate percentage relative error.
In summary, calculating percentage relative error involves finding the difference between the measured and actual values, dividing that difference by the actual value, and then multiplying the result by 100.
Understanding the Calculation of Percentage Relative Error
When I was in school, I always struggled with math. One concept that I found particularly difficult was calculating percentage relative error. However, once I understood the basics, it became much easier.
Calculating percentage relative error involves finding the difference between the measured and actual values, dividing that difference by the actual value, and then multiplying the result by 100. Here’s an example to help illustrate:

Let’s say you’re measuring the length of a table that you know is 100 cm long. Your measurement comes out to be 110 cm. The first step is to find the difference between the measured and actual values:
Measured value – Actual value = 110 – 100 = 10
The next step is to divide the difference by the actual value:
10 \ 100 = 0.1
Lastly, multiply the resulting value by 100:
0.1 x 100 = 10%
Therefore, the percentage relative error is 10%.
Examples of Calculating Percentage Relative Error
Calculating percentage relative error can be used in various situations like science experiments, financial analysis, and educational studies. Here are some examples:

Example 1: John is completing a physics experiment and needs to calculate how accurate his measurements are. He knows the actual value of the weight is 50 lbs, and his measured value was 45 lbs. What is the percentage relative error?
Solution: Taking the formula and substituting the values:
Measured value – Actual value = 45 – 50 = -5
Absolute Value of Percentage Error = |(-5/50) x 100%| = 10%
Example 2: Louise invested $1000 in the stock market, but the price of the stock tanked, and she was left with $500. What is the percentage relative error in Louise’s investment?
Solution: Taking the formula and substituting the values:
Measured value – Actual value = 500 – 1000 = -500
Absolute Value of Percentage Error = |(-500/1000) x 100%| = 50%
Key Takeaways about Calculating Percentage Relative Error
Calculating percentage relative error might seem daunting at first, but it’s easy to understand once you break it down into smaller steps. Here are some key takeaways:
- The formula for calculating percentage relative error is: (Measured value – Actual value / Actual value) x 100%
- This calculation is used to determine how accurate a measurement is compared to the actual value.
- The formula is widely used in science and finance fields.
Common Mistakes in Calculating Percentage Relative Error
Although calculating percentage relative error is a simple concept, it’s common to make mistakes. Here are some common mistakes:
- Forgetting to multiply the result of the difference and the actual value by 100 to get the percentage.
- Not using the absolute value when calculating the percentage error.
- Not understanding the difference between relative error and absolute error.
Frequently Asked Questions (FAQs)
Here are some commonly asked questions about how to calculate percentage relative error:
Q1: What is the difference between relative error and absolute error?
A: Relative error compares the size of the error to the size of the measurement, while absolute error is simply the difference between the measured and actual values.
Q2: How is percentage relative error used in financial analysis?
A: Percentage relative error is used in financial analysis to calculate the difference between the estimated returns and actual returns.
Q3: Can percentage relative error be negative?
A: Yes, percentage relative error can be both positive and negative.
Q4: Why is percentage relative error important?
A: Percentage relative error is important because it allows you to determine the level of accuracy your measurement has compared to the actual value.
Conclusion of how to calculate percentage relative error
Calculating percentage relative error is a concept that many people struggle with, but it’s essential for ensuring accuracy in various fields. By understanding the formula and learning about key takeaways and common mistakes, you can calculate percentage relative error with confidence.
Gallery
Relative And Percent Error Formula (examples, Solutions, Worksheets
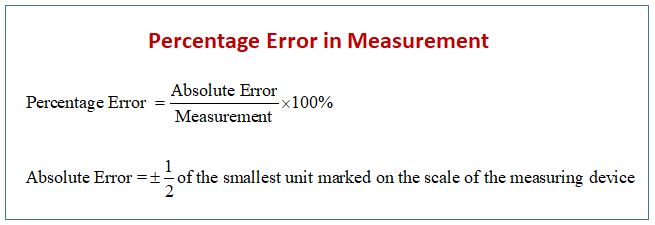
Photo Credit by: bing.com / error percentage formula relative percent measurement absolute
Relative And Percent Error Formula (video Lessons, Examples, Solutions)
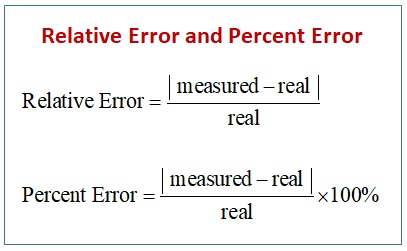
Photo Credit by: bing.com / error relative formula percent examples
Percent Error Calculator – Inch Calculator
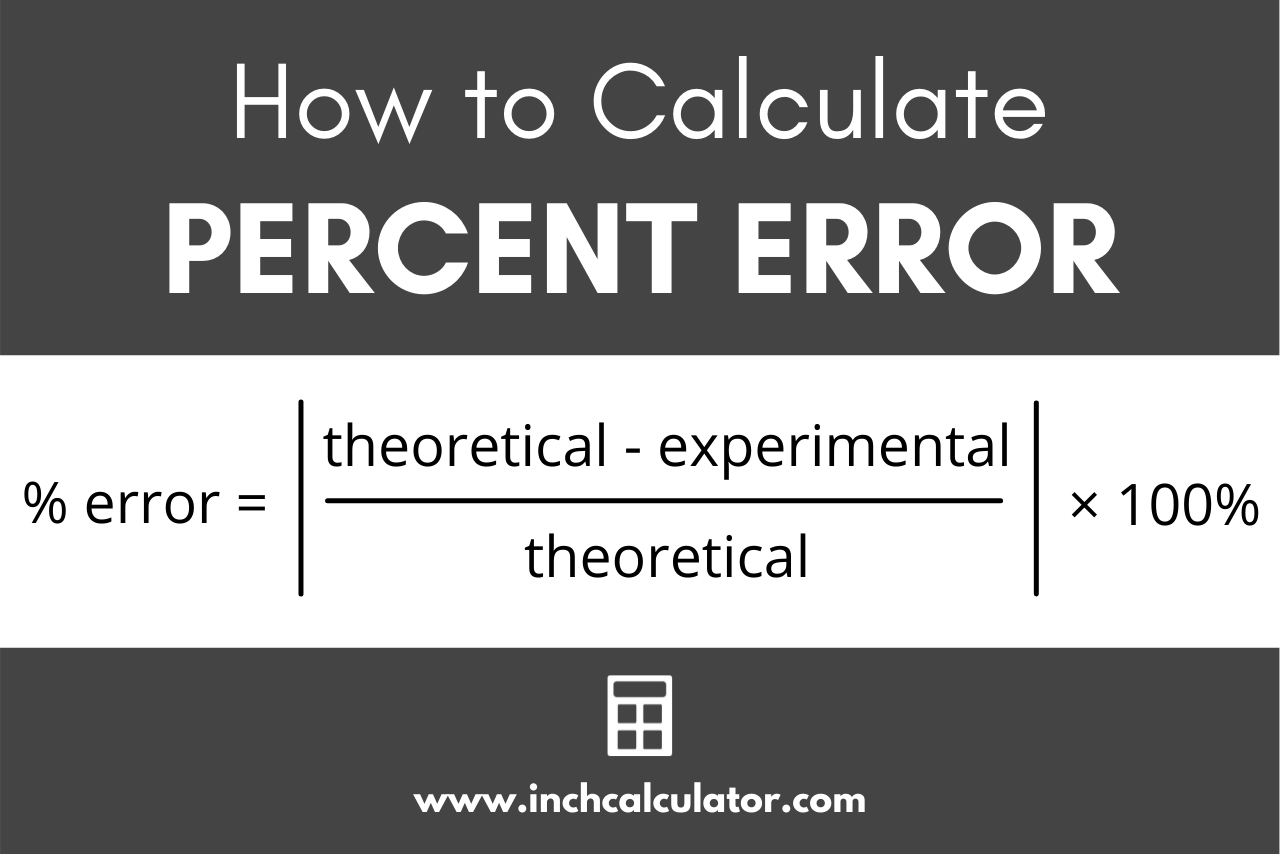
Photo Credit by: bing.com / calculate theoretical inchcalculator minus
Calculate Percent Error Formula – How To Calculate Percent Error : Our

Photo Credit by: bing.com / absolute calculate percentage sciencenotes measurement
How To Calculate Percent Error?- Concept And Calculation, Meaning
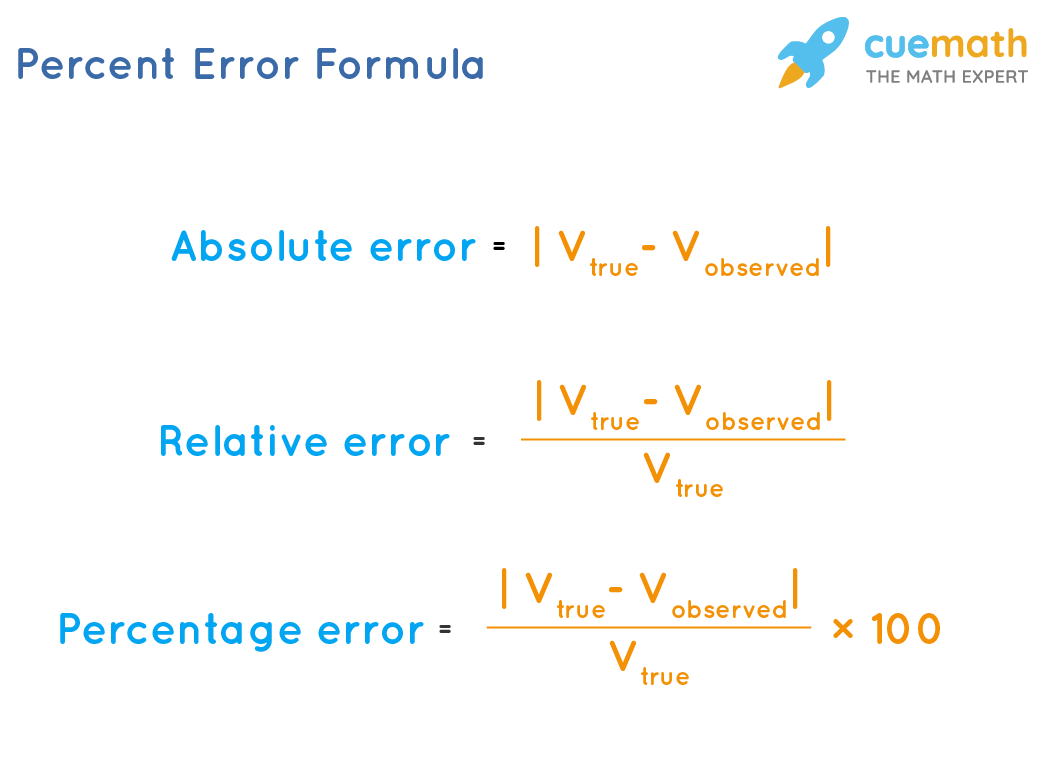
Photo Credit by: bing.com / absolute calculate calculation